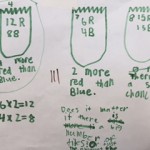Professional Learning Goals

We enhanced our teaching practices in the following ways:

• we adopted and modified Stein and Smith’s five practices for orchestrating mathematics discussions in the classroom

• followed recommendations from formative assessment literature (e.g., Black and Wiliam, 1999) to adjust our in-class teaching actions based on what students shared

•  aligning our practices with inquiry-based learning philosophies (i.e., student-centred methods)

Activities and Resources

We met with graduate students that were enrolled in a mathematics education course from York University. They helped us identify and gain familiarity with the research that related to our professional learning goals. The graduate students also helped us co-plan our lessons by basing the tasks on literature pertaining to geometry, probability and measurement. We opened our classrooms to both teachers and graduate students, video recorded our lessons, and collected student work samples. The graduate students and ourselves analyzed and identified student samples that evidenced mathematical understanding.  Student work samples and video data were analyzed based on the definition that “we understand something if we see how it is related or connected to other things we know” (Hiebert and Carpenter, 1992, p. 4).

Unexpected Challenges

Upon reflection of our entire project experience, we would like to further develop and adapt some of the roles enacted while co-teaching (e.g., the annotator, the whisperer and the questioner). We need more time in each other’s classrooms to refine our process of co-teaching.

We did find it difficult to talk about our classroom practices that were used to elicit and build upon student thinking. The literature is lacking specific nuances of how these practices play out moment-by-moment. We have found some promising research that we would like to explore. We feel that the Towers and Proulx’s (2013) notion of “coordinating the possible” (p. 21) could be useful. Having and developing language would allow us and other teachers to describe and ultimately lead to further enhancements of classroom practices.

Enhancing Student Learning and Development

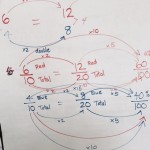
We found evidence of student mathematical understanding in the data we collected – please see student work samples.

Sharing

We have crafted a proposal that we will submit in hopes to present at next year’s OAME conference (2016). We are currently crafting a manuscript (collaborating with graduate students and their instructor) to submit for publication in a peer-reviewed research journal. Our results and experiences have been shared with our colleagues at staff meetings. Sheila Delaney will be the math lead for our school next year, and she is planning on drawing upon our project experiences in her new role.

Project Evaluation

The release time allowed us to spend time doing the math, becoming familiar with related research, discussing a variety of tools and strategies, annotate each other’s thinking, revisit the problem, and provide each other with  feedback on our teaching. This positioned us very well to replicate these math understandings and instructional practices in our classrooms and to reflect on our teaching while we met. We measured the success of our new practices by  identifying students’ mathematical understanding in their work samples.